FISCHER PROJECTION FORMULA:
It is a two-dimensional depiction of an organic molecule at the tetrahedral carbon center. The four bonds at the tetrahedral carbon are represented by two vertical and two horizontal straight lines. The vertical lines are presumed to lie below the plane of the paper and the horizontal lines are presumed to lie above the plane.
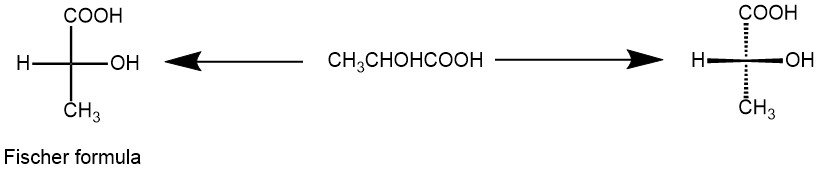
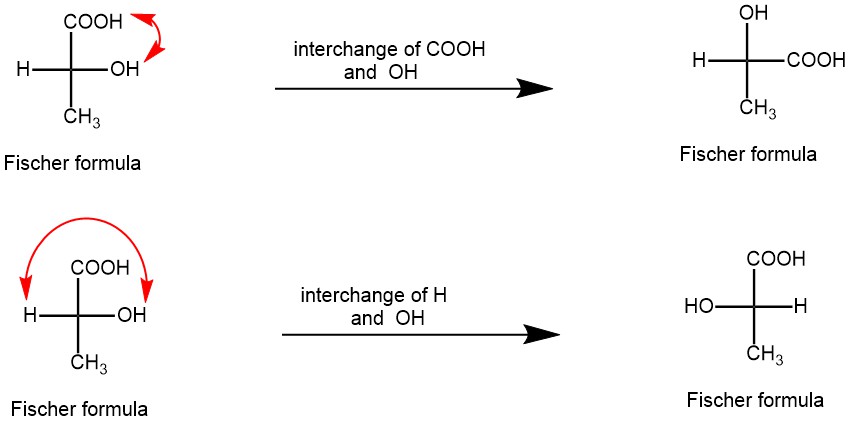
There are certain operations that can be performed on a Fischer projection that retain or change the configuration at the tetrahedral carbon center in the Fischer projection formula.
- Rotation by 180° in a clockwise or anticlockwise direction, without lifting from the plane of the paper, is allowed and this operation does not change the configuration at the chiral center.
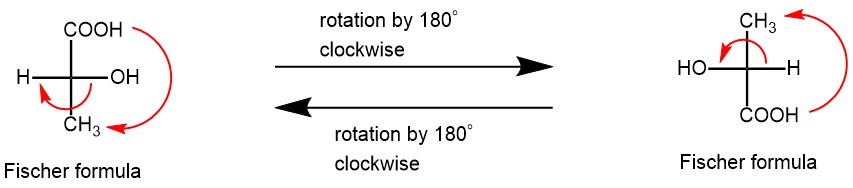
2. Rotation of three groups on the chiral center in a clockwise or anticlockwise direction, without disturbing the fourth group, also does not change the configuration at the chiral center.

3. Rotation of groups in the Fischer formula by 90° changes the configuration at the chiral center.

4. The exchange of any two groups in the Fischer formula by an odd number of times changes the configuration at the chiral center. However, the exchange of two groups by an even number of times does not change the configuration at the chiral center.
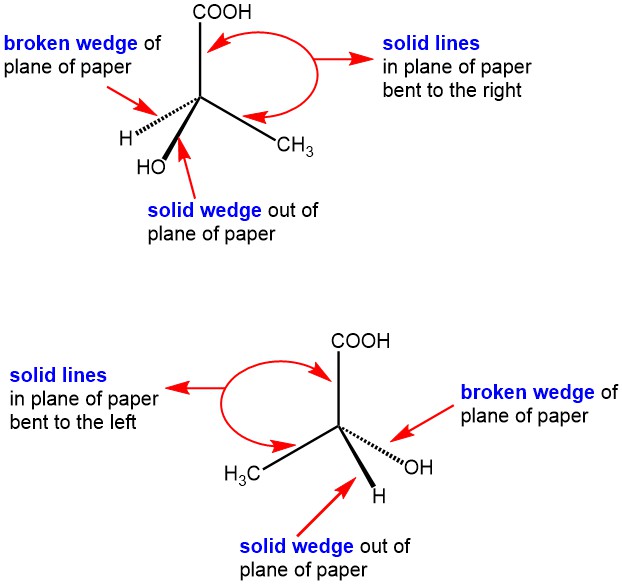
FLYING WEDGE FORMULA:
It is a method of representing the three-dimensional structure of an organic molecule in which two solid lines characterize bonds on the plane of the paper, a solid wedge shows the bond coming out of the plane, and a dashed (broken) wedge shows the bond below the plane of the paper.
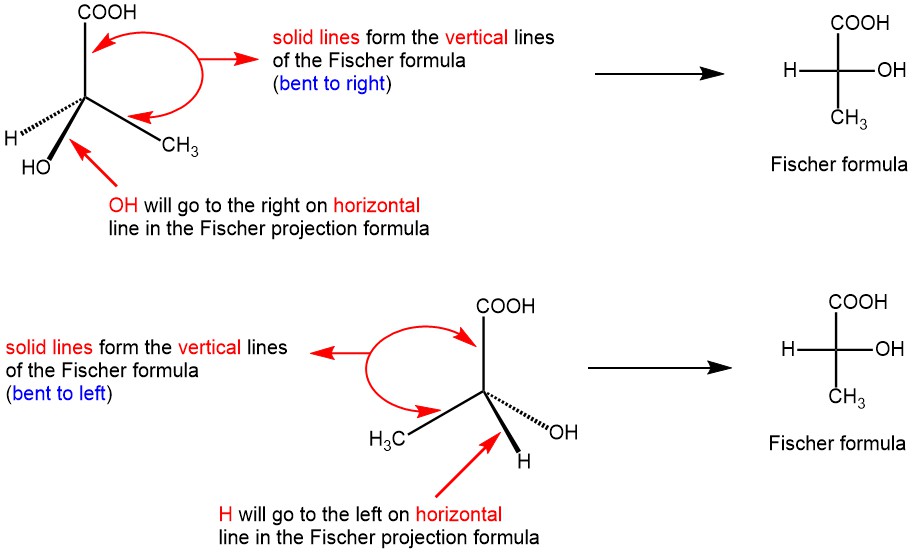
CONVERTING FLYING-WEDGE FORMULA TO FISCHER FORMULA
The two solid lines of the flying-wedge formula are written as the vertical line in Fischer’s formula. The other two groups (on solid wedge and broken wedge) are placed on the horizontal line in Fischer’s formula.
If the two solid lines in the wedge formula are bent to the right, then the group on the solid wedge is placed on the right in the horizontal line of Fischer’s formula.
On the other hand, If the two solid lines in the wedge formula are bent to the left, then the group on the solid wedge is placed on the left in the horizontal line of Fischer’s formula.
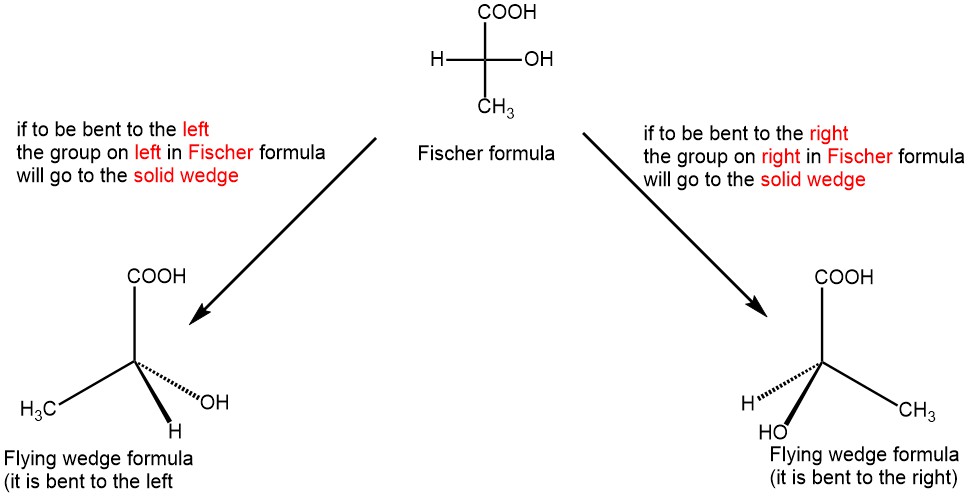
CONVERTING THE FISCHER FORMULA TO FLYING-WEDGE FORMULA
The groups on the vertical line in Fischer’s formula represent two groups on the solid lines in the flying-wedge formula. If the two solid lines are to be bent to the right, then the group on the right in Fischer’s formula will go the solid wedge. On the other hand, If the two solid lines are to be bent to the left, then the group on the left in Fischer’s formula will go the solid wedge.
SAWHORSE PROJECTION FORMULA:
It indicates the spatial arrangement of all the atoms or groups on two adjacent tetrahedral carbon atoms. The two adjacent carbon atoms (indicated by *) are joined by a diagonal line in the plane of the paper, and the remaining bonds on the carbon atoms are shown by smaller lines projected above or below the plane of the paper.

Free rotation along the axis of the two carbon atoms is allowed and this gives rise to different arrangements of the groups in space which are called conformers and the process is called conformation. The structure (conformer) in which the bulky atoms or groups make a dihedral angle q = 180°, the conformation is called as staggered conformation. If the dihedral angle q = 0°, it is called as eclipsed conformation and if the dihedral angle q = 120°, it is called as partially eclipsed conformation.

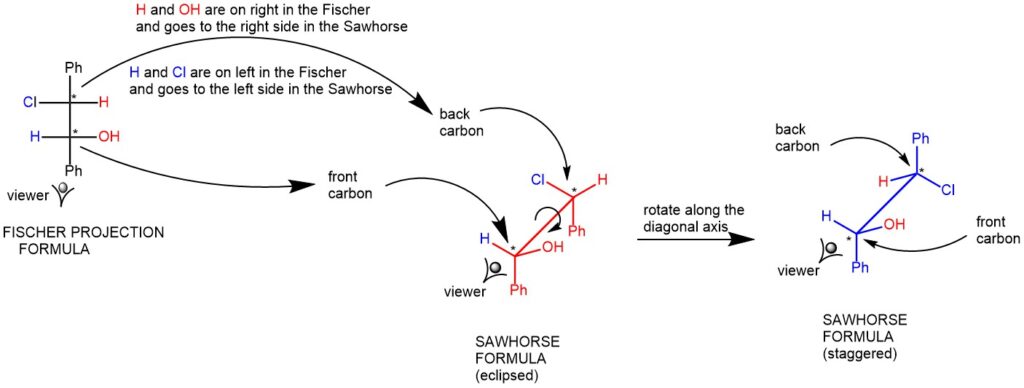
CONVERSION OF SAWHORSE TO FISCHER PROJECTION FORMULA:
The front carbon (closer to the viewer) in the Sawhorse formula forms the lowest carbon in the Fischer formula. For converting the Sawhorse to Fischer formula, the Sawhorse conformation is converted to the eclipsed conformation and the group on the right in the Sawhorse goes to the right and the one on the left in the Sawhorse goes to the left in the Fischer projection formula.

CONVERSION OF FISCHER PROJECTION TO SAWHORSE FORMULA:
The Fischer formula always represents the eclipsed Sawhorse formula and the groups on the right in the Fischer formula go to the right in the Sawhorse formula and the groups on the left in the Fischer formula go to the left in the Sawhorse formula. The eclipsed Sawhorse formula can then be converted to the staggered Sawhorse formula by the rotation along the diagonal line.